My research concerns the mathematical aspects of classical and quantum field theory, with a particular emphasis on gravity (general relativity). On the physics side, I am interested in the formulation of quantum gravity as a field theory, focusing on the problems of causality and observables in quantum gravity. I am also interested in various mathematical topics that play a role in my work, including the following: geometry of PDEs, jet bundles, conservation laws, symmetries, symplectic and Poisson geometry, homological algebra, supergeometry, infinite dimensional analysis, synthetic differential geometry, category theory.

I am a co-organizer of the Prague-Hradec Králové seminar on Cohomology in algebra, geometry, physics and statistics (, recorded lectures). In 2017, I have helped organize the mini-symposium QFT Day in Milan: mathematical aspects of renormalization (poster PDF, SVG). In 2014–2015, I had helped organize the Mathematical Physics Seminar in Trento. During 2016–2019 it was reprised here here.

Contact

Address: Igor Khavkine
Institute of Mathematics CAS
Žitná 25
110 00   Praha 1
Czech Republic
Email: khavkine@math.cas.cz
Web: https://users.math.cas.cz/~khavkine/

Last updated: 14 Oct 2024

Curriculum Vitae

Full CV as a PDF file.

Habilitation: Docent (Geometry & Analysis) Czech Republic
Abilitazione: II Fascia – 01/A4, MAT/07 (Fisica Matematica) Italy
Qualification: MCF25 (Mathématiques Pures) France
Staff Researcher:
2017– 		Department of Algebra, Geometry and Mathematical Physics at the Institute of Mathematics of the Czech Acadey of Sciences (Prague, Czech Republic).
Postdoc:
2016–2017 		Researcher at the Department of Mathematics, University of Milan (Statale).
Postdoc:
2016–2016 		Researcher at the Department of Mathematics, University of Rome 2 (Tor Vergata).
Postdoc:
2013–2015 		Researcher at the Department of Mathematics, University of Trento.
Postdoc:
2009–2013 		Researcher at the Institute for Theoretical Physics, Utrecht University, in the former Quantum Gravity group of Dr. Renate Loll. Funding: NSERC PDF, NWO VENI fellowship.
PhD:
2004–2008 		Degree in Applied Mathematics and Theoretical Physics from The University of Western Ontario, under the supervision of Dr. J. Daniel Chirstensen. Title: Computer simulation of spin foam models of quantum gravity
MSc:
2002–2004 		Degree in Theoretical Physics from The University of Toronto, under the supervision of Dr. Hae-Young Kee. Title: Formation of electronic nematic phase in interacting systems
BSc:
1999–2002 		Degree in Theoretical Physics from Concordia University, Montreal.

Papers

Essentially all of my papers are available from the arXiv preprint server.

Preprints and in preparation

Published

  1. Closed conformal Killing-Yano Initial Data
    A. García-Parrado, I. Khavkine
    Class Quant Grav 39 105002 (2022) [arXiv, doi]
  2. On well-posedness and algebraic type of the five-dimensional charged rotating black hole with two equal-magnitude angular momenta
    M.B. Fröb, I. Khavkine, T. Málek, V. Pravda
    Eur Phys J C 82 215 (2022) [arXiv, doi]
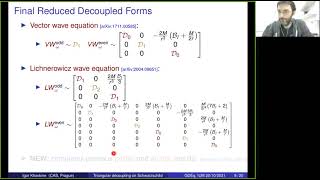
  3. Explicit triangular decoupling of the separated Lichnerowicz tensor wave equation on Schwarzschild into scalar Regge-Wheeler equations
    I. Khavkine
    SIGMA 18 011 (2022) [arXiv, doi]
  4. Compatibility complex for black hole spacetimes
    S. Aksteiner, L. Andersson, T. Bäckdahl, I. Khavkine, B. Whiting
    Commun Math Phys 384 1585 (2021) [arXiv, doi]
  5. Reducing triangular systems of ODEs with rational coefficients, with applications to coupled Regge-Wheeler equations
    I. Khavkine
    Diff Geom Appl 70 101632 (2020) [arXiv, doi]
  6. Conformal Killing Initial Data
    A. García-Parrado, I. Khavkine
    J Math Phys 60 122502 (2019) [arXiv, doi]
  7. Compatibility complexes of overdetermined PDEs of finite type, with applications to the Killing equation
    I. Khavkine
    Class Quant Grav 36 185012 (2019) [arXiv, doi]
  8. IDEAL characterization of higher dimensional spherically symmetric black holes
    I. Khavkine
    Class Quant Grav 36 045001 (2019) [arXiv, doi]
  9. On Wick polynomials of boson fields in locally covariant algebraic QFT
    I. Khavkine, A. Melati, V. Moretti
    Ann H Poincaré 20 929 (2019) [arXiv, doi]
  10. Approaches to linear local gauge-invariant observables in inflationary cosmologies
    M.B. Fröb, T.-P. Hack, I. Khavkine
    Class Quant Grav 35 115002 (2018) [arXiv, doi]
  11. Explicit triangular decoupling of the separated vector wave equation on
    Schwarzschild into scalar Regge-Wheeler equations
    I. Khavkine
    J Phys: Conf Ser 986 012006 (2018) [arXiv, doi]
  12. IDEAL characterization of isometry classes of FLRW and inflationary spacetimes
    G. Canepa, C. Dappiaggi, I. Khavkine
    Class Quant Grav 35 035013 (2018) [arXiv, doi]
  13. Ground state for a massive scalar field in BTZ spacetime with Robin boundary conditions
    F. Bussola, C. Dappiaggi, H.R.C. Ferreira, I. Khavkine
    Phys Rev D 96 105016 (2017) [arXiv, doi]
  14. The Calabi complex and Killing sheaf cohomology
    I. Khavkine
    J Geom Phys 113 131 (2017) [arXiv, doi]
  15. Cohomology with causally restricted supports
    I. Khavkine
    Ann H Poincaré 17 3577 (2016) [arXiv, doi]
  16. Analytic dependence is an unnecessary requirement in renormalization of locally covariant QFT
    I. Khavkine, V. Moretti
    Commun Math Phys 344 581 (2016) [arXiv, doi]
  17. Local and gauge invariant observables in gravity
    I. Khavkine
    Class Quant Grav 32 185019 (2015) [slides, arXiv, doi]
  18. Recurrence relation for the 6j-symbol of suq(2) as a symmetric eigenvalue problem
    I. Khavkine
    Int J Geom Methods Mod Phys 12 1550117 (2015) [arXiv, doi]
  19. Algebraic QFT in curved spacetime and quasifree Hadamard states: an introduction
    I. Khavkine, V. Moretti
    Book chapter in Advances in Algebraic Quantum Field Theory, R. Brunetti, C. Dappiaggi, K. Fredenhagen, J. Yngvason (eds.) (Springer, 2015) [arXiv, doi]
  20. Topology, rigid cosymmetries and linearization instability in higher gauge theories
    I. Khavkine
    Ann H Poincaré 16 255 (2015) [arXiv, doi]
  21. Covariant phase space, constraints, gauge and the Peierls formula
    I. Khavkine
    Int J Mod Phys A 29 1430009 (2014) [arXiv, doi]
  22. Quantum astrometric observables II: time delay in linearized quantum gravity
    B. Bonga, I. Khavkine
    Phys Rev D 89 024039 (2014) [arXiv, doi]
  23. Presymplectic current and the inverse problem of the calculus of variations
    I. Khavkine
    J Math Phys 54 111502 (2013) [arXiv, doi]
  24. Quantum astrometric observables I: time delay in classical and quantum gravity
    I. Khavkine
    Phys Rev D 85 124014 (2012) [arXiv, doi]
  25. Comment on `Hawking radiation from fluctuating black holes'
    I. Khavkine
    Class Quant Grav 28 038001 (2011) [arXiv, doi]
  26. Coupling a Point-Like Mass to Quantum Gravity with Causal Dynamical Triangulations
    I. Khavkine, R. Loll, P. Reska
    Class Quant Grav 27 185025 (2010) [arXiv, doi]
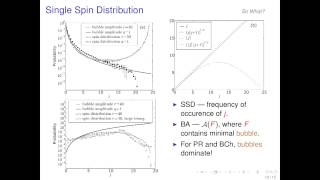
  27. Sub-leading asymptotic behaviour of area correlations in the Barrett-Crane model
    J.D. Christensen, I. Khavkine, E.R. Livine, S. Speziale
    Class Quant Grav 27 035012 (2010) [arXiv, doi]
  28. Evaluation of new spin foam vertex amplitudes
    I. Khavkine
    Class Quant Grav 26 125012 (2009) [arXiv, doi]
  29. Dual Computations of Non-abelian Yang-Mills on the Lattice
    J.W. Cherrington, J.D. Christensen, I. Khavkine
    Phys Rev D 76 094503 (2007) [arXiv, doi]
  30. q-Deformed spin foam models of quantum gravity
    I. Khavkine, J.D. Christensen
    Class Quant Grav 24 3271 (2007) [arXiv, doi]
  31. Supercurrent in Nodal Superconductors
    I. Khavkine, H.-Y. Kee, K. Maki
    Phys Rev B 70 184521 (2004) [arXiv, doi]
  32. Formation of Electronic Nematic Phase in Interacting Systems
    I. Khavkine, C.-H. Chung, V. Oganesyan, H.-Y. Kee
    Phys Rev B 70 155110 (2004) [arXiv, doi]
  33. Strong-field molecular alignment for quantum logic and quantum control
    E.A. Shapiro, I. Khavkine, M. Spanner, and M.Yu. Ivanov
    Phys Rev A 67 013406 (2003) [doi]

Talks

geometry of the 5-dimensional charged rotating black hole (thumbnail)
A look at the geometry of the 5-dimensional charged rotating black hole (05 Apr 2022)
Talk given at the Relativity Seminar (slides), Charles University, Prague. Based on the paper arXiv:2112.13266 with M.B.Fröb, T.Málek and V.Pravda. We studied the algebraic type and regularity, at the horizon and at asymptotically flat null infinity, of the 5-dimensional charged rotating black hole with equal rotation parameters. This solution of the Einstein-Maxwell equations is known in the literature but only numerically.
triangular decoupling on Schwarzschild (thumbnail)
Triangular decoupling of systems of differential equations, with application to separation of variables on Schwarzschild spacetime (20 Oct 2021)
Talk given at the Geometry of Differential Equations Seminar (slides, ), Independent University of Moscow (online). Based on the sequence of works arXiv:1711.00585, 1801.09800, 2004.09651.
homotopy transfer for currents and symmetries (thumbnail)
Homotopy transfer for conserved currents and rigid symmetries in gauge theories (7 Sep 2021)
Talk (slides) given at the Thematic Programme on Geometry for Higher Spin Gravity: Conformal Structures, PDEs, and Q-manifolds (23 Aug – 17 Sep 2021), Erwin Schödinger Institute, Vienna. An informal report on work in progress on extending Noether's theorem in Lagrangian gauge field theory to a homotopy transfer of L∞-algebra structures between symmetries and conserved currents.
analytic structures (thumbnail)
The geometry of analytic structures (17 Aug 2021)
Talk (slides) given at A finite and infinite-dimensional meeting on Lie groupoids, Poisson geometry and integrability (16–20 Aug 2021), Wolfgang Pauli Institute, Vienna. An informal report on work in progress on the geometric interpretation of a real analytic atlas as a G-structure of infinite order.
Finite renormalizations (thumbnail)
Finite renormalizations in locally covariant perturbative algebraic QFT (27 May 2021)
Talk given at the Prague Mathematical Physics Seminar (slides), Charles University (online). Mostly an introductory overview of the topic, with a quick mention of the results from the joint works arXiv:1411.1302, 1710.01937 with V. Moretti and A. Melati.
Kerr compatibility complex (thumbnail)
Killing compatibility complex on Kerr spacetime (16 Dec 2020)
Talk given at the Geometry of Differential Equations Seminar (slides, ), Independent University of Moscow (online). Based on the joint work arXiv:1910.08756 with S. Aksteiner, L. Andersson, T. Bäckdahl, and B. Whiting.
Synthetic PDEs (thumbnail)
A Synthetic Approach to the Formal Theory of PDEs (17 Feb 2017)
Talk given at the Differential Geometry Seminar, Charles University, Prague. Based on the joint work arXiv:1701.06238 with Urs Schreiber.
Projet de recherche (thumbnail)
Présentation du projet de recherche (May 2016)
A presentation (slides) of selected aspects and projects from my research program. (in French)
QG: exercise (thumbnail)
Gravity: an exercise in quantization (31 May 2013)
Talk (slides) given at the Quantum Gravity in Perspective workshop (31 May – 1 Jun 2013), Munich, Germany.
time delay (thumbnail)
Time delay in classical and quantum gravity (13 Jan 2013)
Talk (slides) given at the UC Davis Joint Theory Seminar, Davis, USA.
q-deformed (thumbnail)
q-deformed spin foams for Riemannian quantum gravity (26 Jun 2007)
Talk (slides) given at the LOOPS'07 conference (25 – 30 Jun 2007), Morelia, Mexico.

More

My MathOverflow profile.

clock (thumbnail)
a clock seen through the quantum gravitational vacuum
A visualization of the phenomenon tackled in the papers on the influence of the quantum gravitational vacuum on astrometric observables (time delay, angular position, image distortion). Not to scale! This is an artistst's conception... where the artist is also the scientist. ;)
Zorn's lemma A whimsical illustration of the hypotheses and conclusion of Zorn's Lemma.
polar duality Polar or convex duality maps hyperplanes in a vector space to points of the dual space. Thus, any figure can be mapped to its dual, its envelope of tangents. In particular, the unit balls of p-norm and q-norms (1/p + 1/q = 1) are dual to each other. Click to play around with a Java applet or to see animations for p=1 or p=∞.
temperature vs. coldness A simple Java applet to illustrate the idea of negative temperature. For some special systems, adding heat, past a certain point, will make the temperature climb up to +∞ and then jump to -∞. The basic idea is that, while the temperature T changes discontinuously, the physically more natural parameter coldness β = 1/T remains continuous.